Different methods of expressing the concentration of solution


In Chemistry, “Concentration” expresses the amount of solute present in the solution. Suppose you have been given two jars containing lemon juice and added different quantities of sugar. How will you express its concentration? You will say lemon juice in one jar is “too sweet” and “less sweet” in another. But Chemistry doesn’t use the word “too” to express concentrations. We have to define it.

A solution in which a relative amount of solute is less is known as a dilute solution, and one with a large amount of solute is known as a concentrated solution. But these are the relative terms and do not give quantitative information about the solution. Thus, in this article, we will look at the different methods of expressing the concentration of solutions.

So, let’s start!

1)     Solution concentration or strength:

It describes the amount of solute present in one litre of solution. It is denoted by C or S, and its unit is g/L. It is also known as the strength of the solution.

For example: If 5 grams of salt is added to 0.5 L water, then the concentration of the solution will be  5/0.5 = 10 g/L.

 

2)     Concentration in parts per million (ppm):

It describes the part of a particular component per million (106) parts of the solution. It is expressed as milligrams of solute present in one litre of solution, i.e., mg/L. Another unit for ppm is μg/mL.

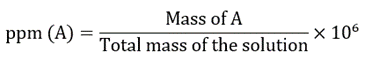
There are some more units used to express concentration in parts: parts per billion (ppb, parts per 109) and parts per trillion (ppt, parts per 1012) and parts per quadrillion (ppq, parts per 1015).

 

3)     Mass/weight percentage or Weight/mass percentage (w/w):

The mass percentage of a solution is the concentration of the solution expressed as the per cent of one component in the solution by mass. Suppose a solution consists of two components:  solute A and solvent B, then the mass percentage of A will be


For example, 30% (w/w) glucose means 30 grams of glucose (solute) is present in 100 grams of the solution, i.e., 30 grams of glucose is dissolved in 70 grams of water.

 

4)     Volume percentage (v/v):

The volume percentage of a solution is the concentration of the solution expressed as the per cent of one component in the solution by volume. Suppose a solution consists of two components:  solute A and solvent B, then the volume percentage of A will be

For example, 20% (v/v) ethanol means 20 mL of ethanol is present in 100 mL of the solution, i.e., 20 grams of ethanol is mixed with 80 mL of water.

5)     Mass by Volume percentage (w/v):

It means the mass of a solute dissolved per 100 mL of the solution. This phenomenon is majorly used in the pharmaceutical industry.

 


6)     Molarity (M):

Molarity is known as the number of moles of solute present in one litre of the solution. It is denoted by ‘M’, and its unit is ‘Molar’ or ‘mol/L’. A mole is the amount of the substance that contains 6.022 X1023 entities, like particles, atoms, ions, molecules, etc., of the given substance. Molarity depends on the temperature.



Suppose a solution of sucrose is marked as 0.5 M. This means that 0.5 moles of sucrose are dissolved in one litre of the solution.

 

7)     Molality (m):

The number of moles of solute dissolved in one kg of the solvent is known as its Molality. It is denoted by ‘m’, and its unit is ‘molal’ or ‘mol/kg’. It is independent of temperature.

 

Suppose a NaCl solution is marked as 0.25 m; this means that 0.25 moles of NaCl are dissolved in one kg of the solvent.

 

8)     Normality (N):

Normality is the number of gram equivalents of solute dissolved in one litre of the solution. Normality is denoted by N, and its unit is ‘Normal’ or ‘equivalents/L’.


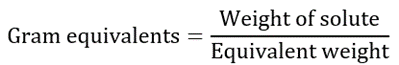
9)     Mole fraction:

Mole fraction is the ratio of moles of one component to the total moles of all the components present in the solution. Suppose we have a solution containing solute A and solvent B. Let nA and nB be solute A and solvent B moles, respectively. So, the mole fractions, xA and xB, of A and B, respectively, can be written as 



10) Formality:

The number of gram formula units present per litre of solution is known as a formality and is denoted by F. When formula mass equals the molecular mass, formality equals the molarity. Formality is useful for ionic compounds in which the molecule does not exist.

 

Conclusion:

In stoichiometric calculations, determining the number of moles and concentrations is important. For preparing solutions during practicals, it is necessary to calculate the weight of the solute that must be weighed or measured. Thus, the methods of expressing concentrations discussed so far are critical.




Writer

- Ajay Shende

- Subject Matter Expert (Chemistry)

- LinkedIn Profile

 

 

Comments

  1. NoahFebruary 27, 2023 at 1:41 PM

    Your idea about simplifying concentration of solution is very effective. Private tutor St. Augustine Thanks for sharing.

    ReplyDelete
  2. Naples TutoringMarch 2, 2023 at 9:56 AM

    Thanks for telling us the different methods Private tutor Ocala

    ReplyDelete

Post a Comment

Popular posts from this blog

The Power of Simulation: Exploring Virtual Experiments in Education

Image
  In today's rapidly advancing technological landscape, virtual simulation has emerged as a powerful tool in the field of education, revolutionizing the way we learn and experience various subjects. One area where virtual simulation has had a significant impact is in the domain of science education. Virtual experiments offer students an immersive and interactive learning experience that transcends the limitations of traditional classrooms. In this blog, we will explore the transformative power of simulation and delve into the world of virtual experiments in education. Understanding Virtual Experiments : Virtual experiments refer to interactive, computer-based simulations that replicate real-life laboratory scenarios. These simulations allow students to engage in hands-on learning without the need for physical apparatus, chemicals, or lab setups. Virtual experiments offer a safe and cost-effective alternative to traditional laboratory experiences by leveraging cutting-edge technolog...
Read more

6 Effective Ways to Study Organic Chemistry

Image
Do you think that organic chemistry is difficult to learn, then you're mistaken. Students memorize reactions one after another, but it leads nowhere. Organic chemistry is the logical subject of chemistry. When you start from the basics and study in an organized way, it makes more sense and gets interesting. So, are you preparing for competitive exams? Here are the six quick and effective ways to learn organic chemistry. Let’s begin your journey by mastering the subject to gain better results in board and competitive exams.   1.      Identify your weak topics and give them a high priority Organic chemistry in the 11 th and 12 th standard books covers the topics essential for advanced learning. Check the syllabus before starting the study of organic chemistry. Due to the many reactions and mechanisms in Organic Chemistry, students may find some lessons difficult. Identify the lessons that you find difficult and important for competitive exams. Give t...
Read more

Digital Reactions: The Rise of Virtual Chemistry Experimentation

Image
  Chemistry is a fascinating and ever-evolving science. For centuries, chemists have relied on physical laboratories to conduct experiments to learn more about the properties of matter and the laws of nature. However, with the rapid advancement of technology, a new era of chemistry experimentation has emerged — virtual chemistry experimentation. This revolutionary approach to conduct chemical reactions in a digital environment is transforming the way we learn, research, and innovate in the field of chemistry. This blog will explore the features and benefits of performing chemistry experiments in a virtual environment. The Evolution of Chemistry Experimentation: Chemistry  experimentation has come a long way from its earliest roots in alchemy. The scientific revolution of the 17th century marked the formalization of experimental methods and the establishment of scientific principles. Since then, physical laboratories have been the focal point of chemistry education, providing h...
Read more